important algebraic formulas, along with examples and their applications in daily life

Algebra is a branch of mathematics that deals with symbols, variables, and the rules for manipulating them. Here are some important algebraic formulas, along with examples and their applications in daily life:

1. Linear Equations:
   • Formula: ax + b = cax+b=c
   • Example: 2x + 3 = 72x+3=7
   • Application: Linear equations are used in various real-life scenarios, such as calculating costs, determining distances, and solving problems involving rates of change.
2. Quadratic Equations:
   • Formula: ax^2 + bx + c = 0ax2+bx+c=0
   • Example: 2x^2 + 3x – 5 = 02x2+3x−5=0
   • Application: Quadratic equations are used in physics to describe motion, in engineering for optimization problems, and in business for profit and loss analysis.
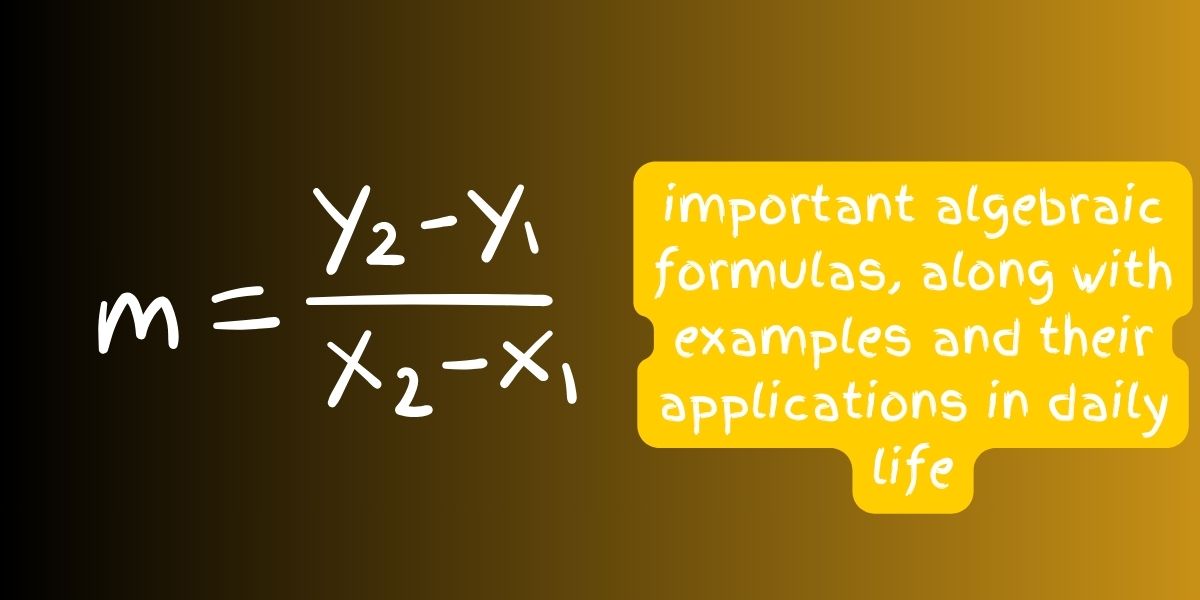
3. Distance Formula:
   • Formula: d = \sqrt{(x_2 – x_1)^2 + (y_2 – y_1)^2}d=(x2​−x1​)2+(y2​−y1​)2​
   • Example: Finding the distance between two points (x1, y1) and (x2, y2) on a coordinate plane.
   • Application: It is used in navigation, surveying, and map-making to calculate distances between two locations.
4. Pythagorean Theorem:
   • Formula: a^2 + b^2 = c^2a2+b2=c2 (for a right triangle)
   • Example: Finding the length of the hypotenuse of a right triangle when the lengths of the other two sides are known.
   • Application: Used in construction, architecture, and engineering for designing structures and ensuring stability.
5. Exponential Growth and Decay:
   • Formula: A = P(1 + r)^tA=P(1+r)t (for exponential growth)
   • Example: Calculating the future value of an investment with compound interest.
   • Application: Used in finance to model investments, population growth, bacterial growth, and radioactive decay.
6. Arithmetic and Geometric Sequences:
   • Formula:
     • Arithmetic: a_n = a_1 + (n – 1)dan​=a1​+(n−1)d
     • Geometric: a_n = a_1 \times r^{n-1}an​=a1​×rn−1
   • Example: Finding the nth term of a sequence with a given first term (a1) and common difference (d) for arithmetic sequences, or common ratio (r) for geometric sequences.
   • Application: Used in finance, computer algorithms, and natural phenomena modeling.

Understanding and applying these algebraic formulas can help solve a wide range of problems in daily life, from managing finances and planning routes to designing structures and analyzing data.